By Stephen Meskin
Because we are elderly, my wife feels it is important for us to downsize. She has felt this way for some time, but at the recent urgings of our governor to stay home, she thinks this is the perfect time. Because she is a great cook and for other personally comforting reasons, I go along with her as long she doesn’t touch my math library.
However, I touch it.
A few days ago, I noticed a very old-looking paperback that might be a candidate for downsizing. When I pulled it out, I discovered that it was a 1958 reprint of a 1919 edition of a book of puzzles by H.E. Dudeney titled The Canterbury Puzzles.
The first quarter of the book consists of puzzles that Dudeney claims were written by Chaucer but left out of Chaucer’s well-known Canterbury Tales. Thus, the name of Dudeney’s book. Dudeney had no problem using Chaucer’s puzzles, so—with a little light editing—I will have no problem either. (See below.)
Needless to say, The Canterbury Puzzles will not be part of our downsizing.
The Steward’s Puzzle
Chaucer’s term for Steward was Manciple, a person who purchased victuals (food) for institutions. The Steward who accompanied the travelling party was a wily man, who had more than 30 masters and made fools of them all. Yet he was a man whom purchasers might take as an example of how to be wise in buying food.
One day during the journey, the Miller and the Weaver sat down to a light repast. The Miller produced five loaves and the Weaver three. The Steward, coming upon the scene, asked permission to eat with them, to which they agreed. When the Steward finished eating, he laid down eight coins and said with a sly smile, “Settle betwixt yourselves how the money shall be divided. ’Tis a riddle for thy wits.”
Solve the riddle assuming that all three ate the same amount of bread.
The Miller’s Puzzle
The Miller showed the party nine sacks of flour that were standing as depicted in the sketch. “Hearken,” said he, “while I set ye the riddle of the nine sacks of flour. Mark ye, that there be single sacks on the outside, pairs next unto them, and three together in the middle. It so happens that if we multiply the pair, 28, by the single one, 7, the answer is 196, which is the number shown by the sacks in the middle. Yet when the other pair, 34, is multiplied by its neighbor, the answer is not 196.

Wherefore, I beg you, gentle sirs, to place anew the nine sacks so that each pair when multiplied by its single neighbor shall make the number in the middle.”
There is more than one solution; find them all. The Miller further stipulated that as few bags as possible be moved—in this case the solution is unique; find it.
The Pardoner’s Puzzle
(In Chaucer’s time a Pardoner was an itinerant fundraiser for the church and related good works.) The gentle Pardoner, “that straight was come from the court of Rome,” begged to be excused; but the company would not. “Fellow-pilgrims,” said he “the riddle that I have made is but a poor thing, but it is the best that I have been able to devise.” But his invention was very well received. He produced the accompanying plan and said that it represented 64 towns through which he had to pass during some of his pilgrimages, and the lines connecting them were roads. He explained that the puzzle was to start from the large red town and visit all the other towns once, and only once, in 15 straight pilgrimages. (By a “straight pilgrimage,” he means a rook’s move.) You may end where you like—but note that the omission of the little road at the bottom is intentional, as it seems it was impossible to go that way.
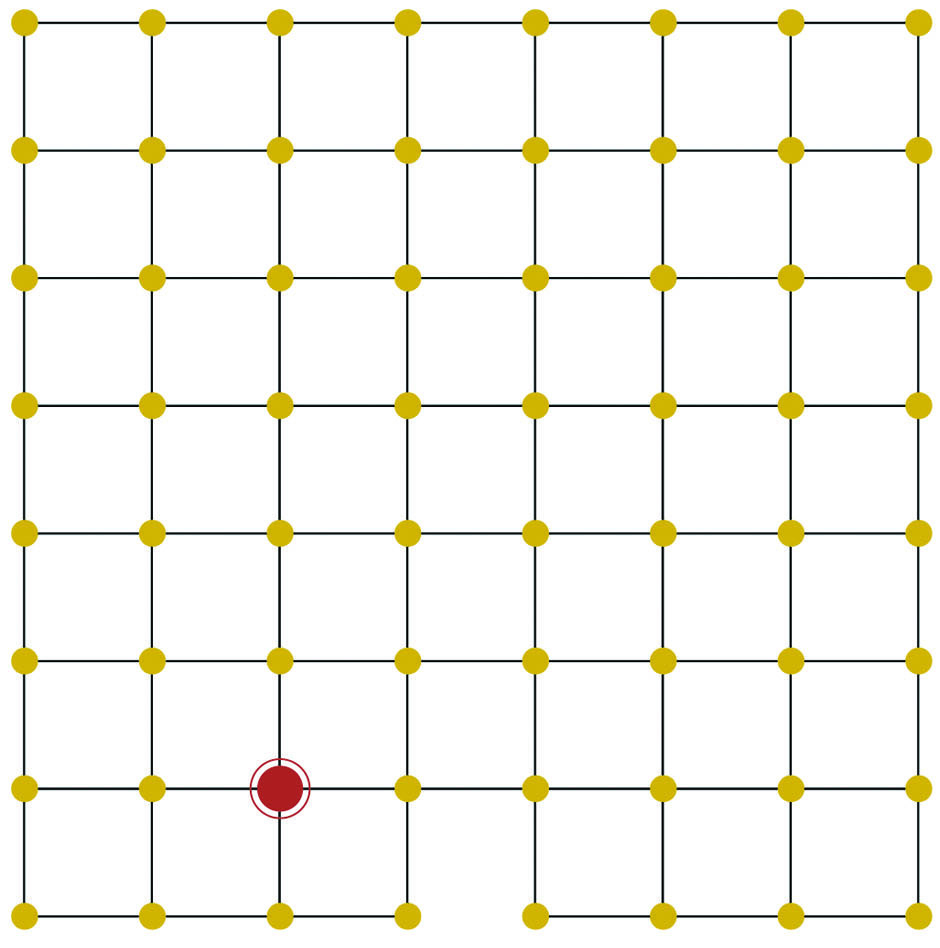
Again, there are multiple solutions, but in this problem, one solution will do.
This puzzle is fascinating. I don’t know a method to find solutions and there are many ways to vary it. What happens if we change the starting point, or require that we end up where we started, or delete more roads, or change the configuration, or change the number of towns? I would enjoy hearing about solutions to any of these or other variations.
Solutions may be emailed to cont.puzzles@gmail.com.
In order to make the solver list, your solutions must be received by May 31, 2020.
Solutions—Take Your Vitamins
1. What is the probability of a perfect bottle without cheating? With 28 vitamins, note that it is impossible to obtain a perfect bottle if 14 or more of the 28 original vitamins are orange. If there are 13 orange vitamins, after taking an orange with a random vitamin 13 times, you are left with 2 non-orange vitamins, each with a 50% chance of being red or purple. If 1 is red and 1 is purple, you win.
We can use the binomial distribution to find the probability of having exactly 13 orange vitamins, 0.0536, and multiply that by 0.5, the probability of having exactly 1 purple vitamin in the remaining 2. The product equals 0.0268. If there are 12 orange vitamins, after taking those 12 oranges with a random color, 4 vitamins remain, and you win if exactly 2 of them are purple. The probability here is 0.0872 × 0.375=0.0327.
Continuing this method for 11, 10, 9 … all the way to 0 orange vitamins, and summing the results leads to the product of a perfect bottle at right around 25.7%.
2. What is the probability of a perfect bottle if you cheat? Again, if there are 14 or more orange vitamins originally, you lose even with cheating. If there are 13 orange vitamins, you can cheat your way to victory as long as you have between 1 and 14 red vitamins. Similarly, if there are 12 orange vitamins, you can cheat your way to a perfect bottle if there are between 2 and 12 red vitamins.
You can continue this process all the way until there are 0 orange vitamins, compute the probabilities via the binomial distribution, and when you sum up everything, you get a perfect bottle probability of 90.7%.
Solvers
Robert Bartholomew, Bob Byrne, Bob Conger, Andrew Dean, Bernie Erickson, Nick Fiechter, Rui Guo, David Kausch, Clive Keatinge, Alex Kozmin, David Lovit, Paul Navratil, David Oakde, David Promislow, John Snyder, Al Spooner.





